In at least one way, parallelograms are extra special: So many other quadrilaterals are also parallelograms. When you learn rules that apply to parallelograms in general, you can assume that these same rules apply to other special quadrilaterals in the parallelogram "family." Furthermore, understanding the relationships among the sides and angles of a parallelogram can help you solve many types of problems.
Let's explore some of the properties of quadrilaterals that are also parallelograms. Answer the question beneath each image. Then click the image to check your answer.
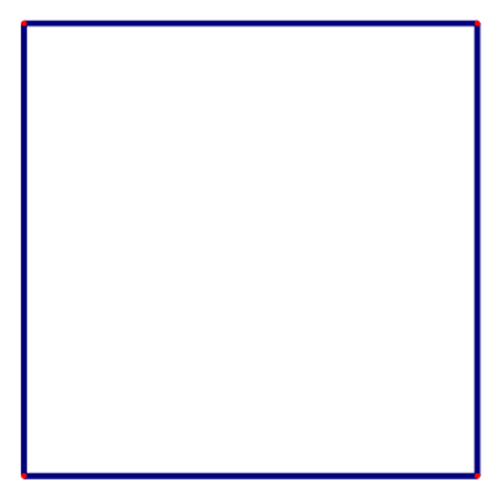 |
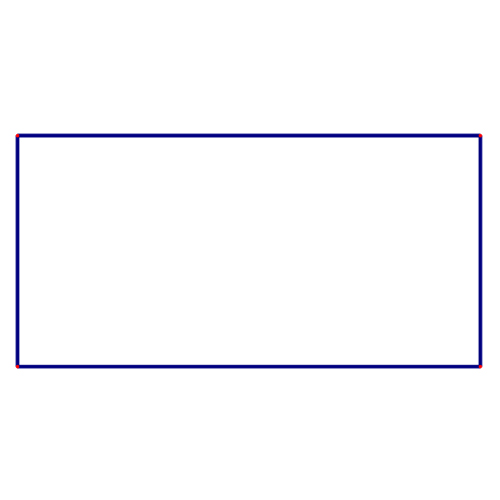 |
| What makes the square a parallelogram? | Why is the rectangle a parallelogram? |
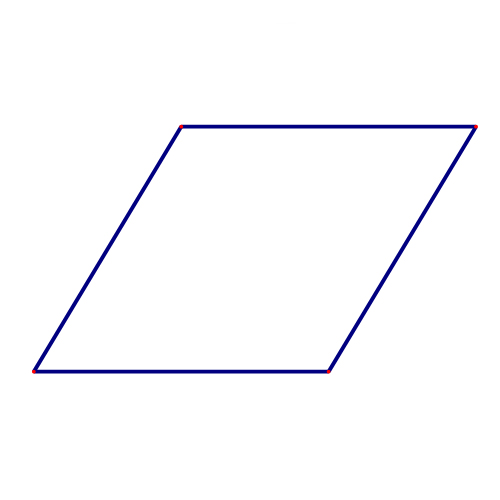 |
 |
| What makes the rhombus a parallelogram? | Why is this quadrilateral a parallelogram? |
Question
Based on these examples, can you name three properties of parallelograms?
1. Parallelograms have all the properties of quadrilaterals.
2. Parallelograms have opposite sides that are parallel.
3. Parallelograms have opposite sides that are congruent.
Because the rules in this lesson apply only to parallelograms, it's important to distinguish parallelograms from other geometric shapes.
