What is the perimeter of this square?
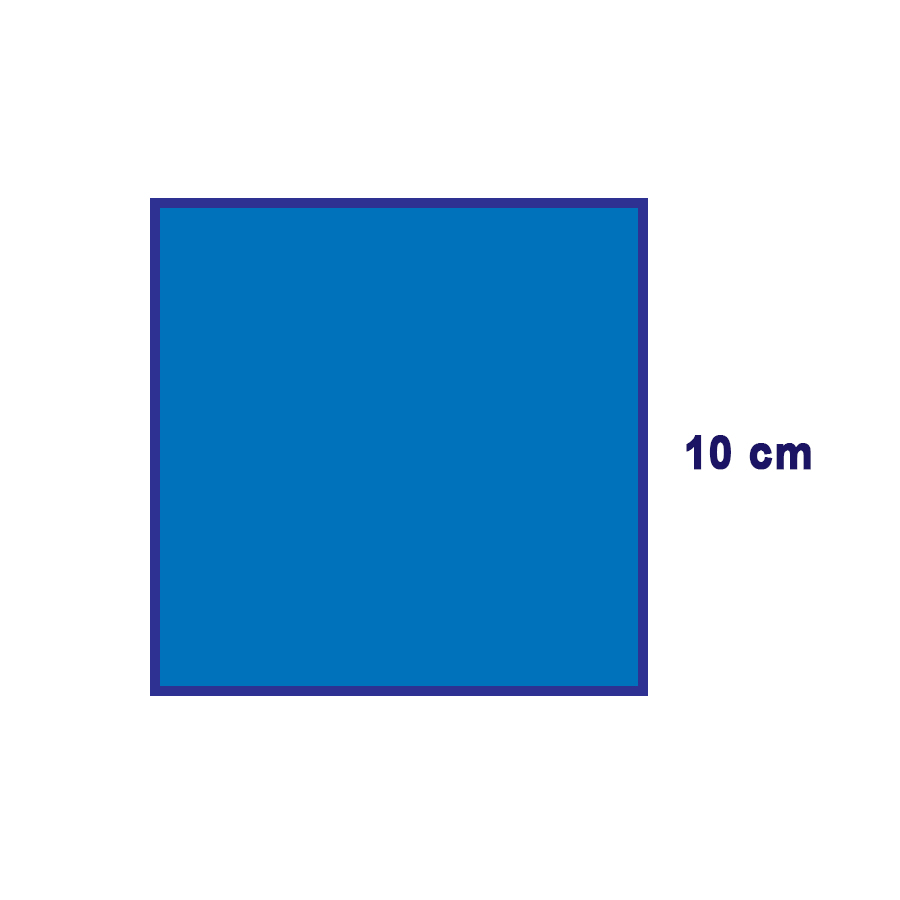
P=(4)(10cm)=40cm
Let's have a look...
You have learned that the perimeter of a figure is the distance around the figure. Look at the red outline of each figure below.
Imagine that you were mowing this square-shaped lawn.
The perimeter is the distance that you would walk around the edge of the lawn.
Imagine that you were trimming the plants in this triangle shaped garden bed.
The perimeter is formed by the plants along the edge of the triangle.
To calculate the perimeter of a shape, find the sum of the lengths of its sides. Click each tab to learn how.
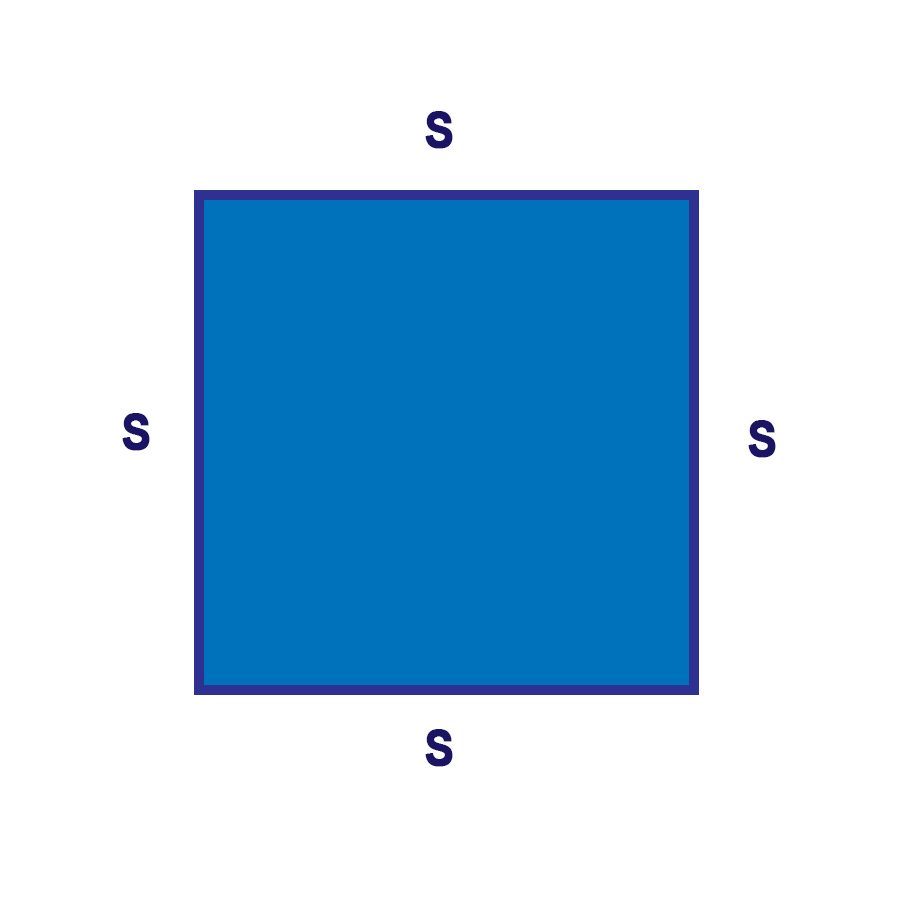
The perimeter (P) of a square is:
P=s+s+s+s
Because a square has four sides of equal length, the perimeter of a square is four times the length of one side. You could calculate the perimeter of a square by multiplying the length of one side by 4. For this square, the length of each side is s. Therefore,
P=4s
If s=2m, then what is the perimeter of this square?
Each side of the square is 2m. Therefore,
P=2m+2m+2m+2m=8m
You could also calculate the perimeter of this square by multiplying the length of one side by 4.
P=(4)(2m)=8m
Let's Practice
Practice calculating perimeter by completing the activity below. Calculate the perimeter of the square or triangle on each slide, then check your answer.
