Later in this course, you will need to draw and label sets of lines in order to solve problems. The slides below will show you how to construct parallel lines. The slides on the next pages will show you how to draw perpendicular lines. You'll need to follow along in your notebook, so grab a pencil, a straightedge, and a compass. These tools are essential for most geometric constructions.
|
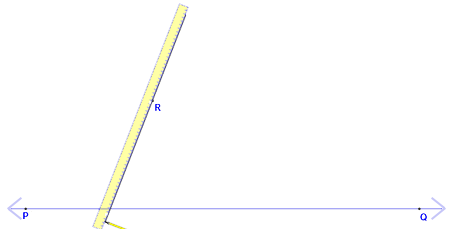
Start by drawing a line PQ anywhere on your paper. Make sure to leave room at the top of the line because you will end up with a "top" line that is parallel. Make a point R not on the line PQ.
Next, you'll take your straightedge and draw a line from point R to a new point J on the line PQ. This new line should be at a slight incline as shown in the picture.
This is what you should have after you finish.
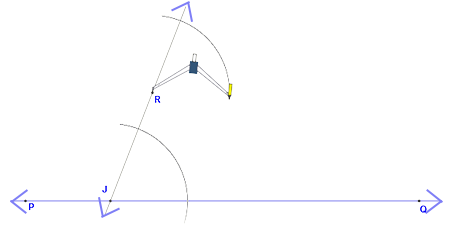
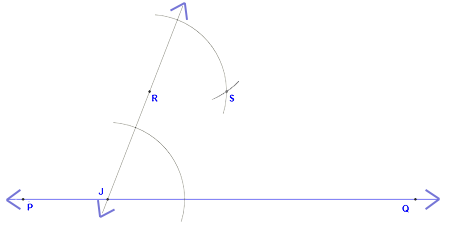
Next, open up your compass to a width so that your arc will intersect angle RJQ. Next, place the metal tip of the compass on the point J and then draw an arc that passes through the lines PQ and RJ. Your arc should intersect angle RJQ as shown.
Now, we will repeat step 3 but beginning at point R. So let's repeat step 3. Do not change the width of the compass. Place your metal tip of the compass at the point R. Then, make an arc that crosses the line RJ.
This is what your page should look like now. Make sure your arcs sweep towards line PQ. Notice that the lower arc crosses line PQ and the upper arc does not cross line PQ.
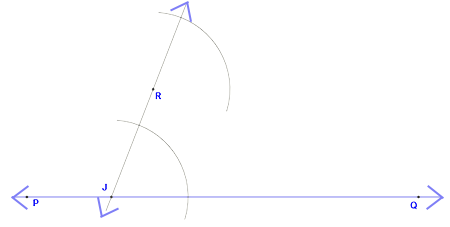
We are almost there! Now, open your compass equal to the distance between the points where the lower arc intersects line JR and line PQ, as shown.
Be careful not to change the width of the compass! Put the metal tip of the compass where the top arc intersects line JR. Make a short arc that crosses with the upper arc. Let's call the point that is formed point S.
This is what you get once this step is done.
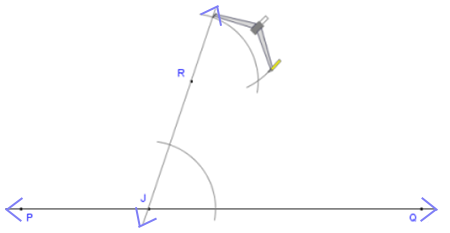
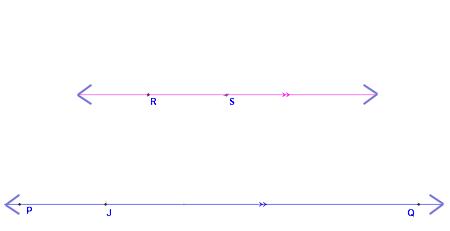
Do you see what your last step would be to get the parallel line? You have to actually draw the line that intersects points R and S. This will be your new line RS, which is parallel to line PQ.
This is the final view of your new parallel lines after the marks made during the construction are removed. However, in a construction problem, you would not remove any construction marks! |